 |
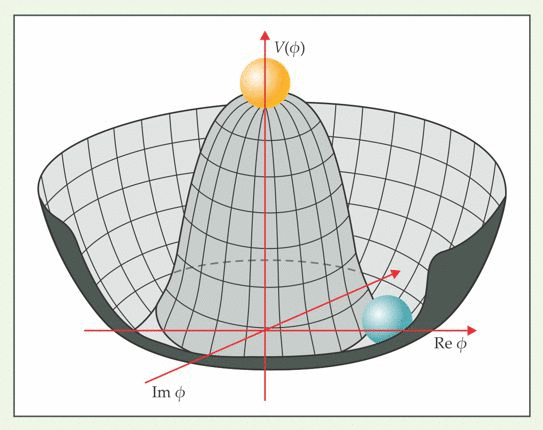
| Figure 2. The Mexican-hat potential energy density considered by Jeffrey Goldstone in his seminal 1961 paper. 2 The energy density is a function of the real (Re) and imaginary (Im) values of a spinless field ϕ. In the context of the electroweak theory developed later in the decade, the yellow ball at the top of the hat would represent the symmetric solution for the potential, in which the photon, W bosons, and Z boson are all massless. The blue ball in the trough represents the solution after symmetry breaking. In that solution the W and Z bosons are massive and the photon remains massless. The steepness of the trough is related to the mass of the Higgs boson. Citation: Phys. Today 66, 12, 28 (2013); http://dx.doi.org/10.1063/PT.3.2212 |
Symmetries and other regularities of the physical world make science a useful endeavor, yet the world around us is characterized by complex mixtures of regularities with individual differences, as exemplified by the words on this page. The dialectic of simple laws accounting for a complex world was only sharpened with the development of relativity and quantum mechanics and the understanding of the subatomic laws of physics. A mathematical encapsulation of the standard model of particle physics can be written on a cocktail napkin, an economy made possible because the basic phenomena are tightly controlled by powerful symmetry principles, most especially Lorentz and gauge invariance.
How does our complex world come forth from symmetrical underpinnings? The answer is in the title of Philip Anderson’s seminal article “More is different.” 1 Many-body systems exhibit emergent phenomena that are not in any meaningful sense encoded in the laws that govern their constituents. One reason those emergent behaviors arise is that many-body systems result from symmetries being broken. Consider, for example, a glucose molecule: It will have a particular orientation even though the equations governing its atoms are rotationally symmetric. That kind of symmetry breaking is called spontaneous, to indicate that the physical system does not exhibit the symmetry present in the underlying dynamics.
It may seem that the above discussion has no relevance to particle physics in general or to the Higgs boson in particular. But in quantum field theory, the ground state, or vacuum, behaves like a many-body system. And just as a particular glucose orientation breaks an underlying rotation symmetry, a nonvanishing vacuum expectation value of the Higgs boson field, as we will describe, breaks symmetries that would otherwise forbid masses for elementary particles. Now that the Higgs boson (or something much like it) has been found at the Large Hadron Collider (LHC; see Physics Today, September 2012, page 12), particle experimentalists are searching for more kinds of Higgs bosons and working to find out if the Higgs boson interacts with the dark matter that holds the universe together. Cosmologists are trying to understand the symmetry-breaking Higgs phase transition, which took place early in the history of the universe, and whether that event explains the excess of matter over antimatter. The measured mass of the Higgs boson implies that the symmetry-breaking vacuum is metastable. If no new physics intervenes, an unlucky quantum fluctuation will eventually spark a cosmic catastrophe.
Physics Today: The future of the Higgs Boson
Comments